
Summary: On price formation theory in Sabiou M. Inoua and Vernon L. Smith Economics of Markets
To suppose that utility maximizing individuals choose quantities to buy (sell) contingent on given prices is to pose a consumer demand and supply problem without a price-determining solution. This neoclassical problem formulation imposes (1) exogenous prices, (2) price-taking behavior, and (3) the law of one equilibrium-clearing price on markets, before prices can have formed. Hence, unexplained prices are presumed to exist before consumers arrive in the market. If conditions (2) and (3) are hypothesized to characterize markets, the theoretical challenge is to show that they follow from a theory of how markets function. Hence, neoclassical economics did not, because it could not, articulate a market price formation process.
The classical economists suffered none of these inconsistencies. (see for example Adam Smith, 1776, book 1, chapter VII) They articulated a coherent theory of price formation and discovery based on operational pre-market assumptions about the decentralized information that buyers and sellers brought to market, their interactive market behavior in aggregating this information, and simultaneously determining prices and contract quantities in the market’s end state.
Buyers (sellers) were postulated as having pre-market max willingness to pay, wtp (min willingness to accept, wta) value (cost) for given desired quantities to purchase (sell) that bounded the price at which each would buy (sell) as they sought to buy cheap (sell dear). We articulate a mathematical theory of this classical price formation process, its connections with
Shannon information theory, and the unexpected role of early experiments in using designs and reporting results consistent with classical theory:
- Individuals go to market with pre-market max wtp (min wta) reservation values (costs) for discrete (integer) units Arriving, they bring aggregate WTP (WTA) conditions governing price bounds, and motivation to buy cheap (sell dear).
- Any trial (bid/offer) price, P, if too low, tends to rise; if too high, tends to fall. Hence, in classical price adjustment, the “law of demand and supply”, is dynamic. Formally, price change and excess demand, e(P), have the same sign: e(P) ΔP/Δt > 0, if e≠ 0; price changes if excess demand is not
- Short side rationing: If any (bid or offer) trial price, P, is too low, the units bought (demanded) are limited by the supply quantity; if P is too high, the units sold (supplied) are limited by the demand quantity. Hence, quantity traded is the minimum of quantity supplied and quantity demanded, or formally, min[s(P), d(P)].
From 2., let V(P) = integral (sum) of -e(P) [namely excess supply, s(p)-d(p)]; for discrete values
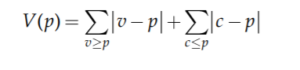
where the notation means summation over all values, v ≥ p, and all costs, c ≤ p, to assure that no goods trade at a loss. Define (the market center of value),
C = arg Min V(P),
which includes market clearing, with C = P* (“equilibrium” price), but is more general, by including important cases like constant cost industries where the exchange quantity is determined by demand.
Notice that V(P), a Lyapunov function, measures the distance between price and the traders’ reservation values in profit space. At any P we have, ΔV/Δt = ─e(P)ΔP/Δdt ≤ 0 where t is transaction number.
Hence, V changes non-positively as transactions increase, a parameter-free law of classical market convergence. To get convergence speed, a quantitative result, we would need institutional parameters relating transactions to calendar time.
For a smooth “large market”, where we let the number of agents increase without bound (infinite),
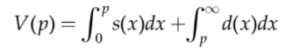
and dV/dt = -e(P)dP/dt ≤ 0.
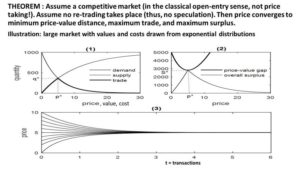
Here is a chart illustrating the above equations for large markets in which all “motion” is in terms of transactions, t ≥ 0 , not time, and is therefore a qualitative dynamics.

READER COMMENTS
Knut P. Heen
Mar 23 2021 at 1:19pm
Suppose each producer produces one unit and takes it to market.
Suppose the number of producers is infinite.
Isn’t the supply infinite?
Where is the scarcity?
Jon Murphy
Mar 23 2021 at 1:49pm
Of course if we suppose there is no scarcity then there is no scarcity. But scarcity comes about because there are unlimited wants and only limited means.
Without scarcity, there is no economic problem. Prices do not exist without scarcity.
Phil H
Mar 24 2021 at 12:12am
While I see the point you’re making, the striking feature of the modern economy is how scarcity can apparently be manufactured. In the UK, water from taps is drinkable; but there is still a market for bottled water. Human imagination is infinite, but there is still a market for the products of imagination.
If prices are in fact the product of negotiation between suppliers and consumers, then it’s not obvious that scarcity is a necessary factor… I dunno if this is an excessively philosophical point, but it seems relevant to the discussion around house prices, for example. It’s not obvious that the scarcity is the thing.
Jon Murphy
Mar 24 2021 at 7:57am
Huh? I don’t understand you. Start here: what is scarcity?
KevinDC
Mar 24 2021 at 2:22pm
Right, but that has nothing to do with “manufactured scarcity.” Bottled water has features that a tap doesn’t have – portability, for example. Some people also claim to find the taste of bottled water better than tap water, although for me this difference is marginal. Tap water is cheaper per unit volume than bottled water, but bottled water is more convenient and (allegedly) better tasting. We don’t need a concept like “manufactured scarcity” to explain why people are willing to buy bottled water despite the existence of taps.
Admittedly pedantic nitpick: human imagination is certainly vast, but I doubt that it’s “infinite” – the human mind, while marvelous, is still a limited tool. But even if it was infinite, that doesn’t mean it’s not also scarce, in the economically relevant sense. In economics, “scarce” does not mean the same thing as “limited” or “rare.” Something can be overflowingly abundant, and still economically scarce. This is a common confusion. I often say, as economists, we are really bad at naming things in a way that makes intuitive sense to non-economists. This often has the side effect that someone who isn’t an economist hears an economic term, thinks they understand what it means in economics, and can end up reading that term in economic discussions in a way that is coherent in their own mind, but incorrect for the purpose of the discussion, and miss the point of the argument. (I.e., trying to argue that the supply of a good has been increasing by pointing to an increase in the quantity supplied for that good – but an increase in supply and increase in quantity supplied are very different things and have very different economic implications.)
The most important cost in economics – really, what all cost boils down to – is opportunity cost. Even if humans had boundless and endless imagination, there would still be opportunity costs associated with the use of imagination, because time spent imagining things and applying the results of that imagination is time spent not doing other things of value. And this opportunity cost for the uses of imagination (or human mental pursuits generally) makes imagination just as subject to scarcity, and the laws of economics, as quartz or corn or vulcanized rubber.
Phil H
Mar 24 2021 at 9:18pm
Yep, I think I basically accept that. It’s a very different point to what Jon was saying, and very different to the old-fashioned conception of economics, as the study of the allocation of scarce resources. In your formulation it’s not the resources that are scarce, it’s the ability to use the resources.
There is, nonetheless, a worry even with your formulation: our ability to consume has increased vastly over the past century. Sitting here in my comfortable flat, tapping on a computer, I’m consuming many more resources, even while doing an activity (writing) that people have been doing for millennia. And one of the tenets of most economists is that our capacity to consume is practically infinite. So even our consumption per hour is not necessarily “scarce” in the long term.
So it’s still not obvious to me that it really is any kind of scarcity that drives prices. What drives prices is negotiation. It doesn’t matter if I’m motivated by scarcity or anything else. As a buyer, I negotiate, and a price gets set. As a seller, I negotiate, and a price gets set.
KevinDC
Mar 25 2021 at 10:09am
Actually, what I said is the “old-fashioned conception of economics.” I wasn’t offering up some new insight that has somehow eluded the rest of the economics profession – truly, I wish I was clever enough to have such insights! But what I said is a pretty humdrum econ 101 level statement that you can find expressed in various forms in any introductory textbook. And it’s entirely consistent with Jon’s statement that “scarcity comes about because there are unlimited wants and only limited means” and that “Without scarcity, there is no economic problem. Prices do not exist without scarcity.”
You also note that “In your formulation it’s not the resources that are scarce, it’s the ability to use the resources.” I think this might be where you’re being misled into thinking there is some gap between what Jon said and what I said, because in economics, this is a distinction without a difference. “Resources” are simply whatever things you have the ability to use (or use productively, to be more precise). Anything you don’t have the ability to use isn’t a resource, by definition. If your ability to make productive use of inputs is scarce, for whatever reason, that is logically equivalent to the statement “resources are scarce” as the terms are defined and used in economics. The best articulation of this point, and its implications, can be found in Julian Simon’s book with the rather on-the-nose title of The Ultimate Resource.
For example, it’s not as if oil was always a resource, and for the vast majority of human history nobody realized it. For most of the existence of our species, it wasn’t a resource, until we developed the capacity to use it productively, and then it became a resource. Many things which exist today that are considered waste or garbage, could change into being resources tomorrow, if we gain the ability to use them. Our capacity to use things is what defines resources. So the statement “It’s not resources that are scarce, it’s the ability to use resources” entails a contradiction, at least considering what “resource” means in economics.
Jon Murphy
Mar 25 2021 at 10:56am
Thanks KevinDC. You expressed the point far better than I could
Phil H
Mar 25 2021 at 8:40pm
Yeah, that doesn’t make any sense. There are two different situations.
(1) “time spent … is time spent not doing other things of value” in this situation, the person has the ability to choose an alternative resource, but doesn’t (because of time constraints)
(2) “Anything you don’t have the ability to use isn’t a resource” In this situation, the person doesn’t have the ability to use a thing (e.g. oil, because the technology hasn’t been invented yet)
These two are logically distinct. Failure to see that is a big problem!
JFA
Mar 26 2021 at 7:47am
Two things can be logically distinct but still be true at the same time. Logically distinct does not mean mutually exclusive. The activity of sitting and the activity of watching TV are distinct but you can still do them at the same time.
In #1, the existence of time constraints and the fact that some activities are mutually exclusive (i.e. cannot be done at the same time) is where the opportunity cost explanation comes in. This is even the case if you had access to an infinite number of goods and resources and no technical inability to use those physical objects. That’s one reason for scarcity (and the one that economist mostly focus on).
In #2, the technical ability to utilize things puts bounds on what is in the set of consumption choices. Just because ancient civilizations had access to petroleum (which is used to make plastic), sand (used to microchips), and various metals doesn’t mean that they had access to computers. Before natural gas was able to be captured, it was not an option as a fuel resource. Before humans figured out that flint could be used to start fire, it was just a rock. All of these are examples of things being scarce.
These are standard Econ 101 concepts. If you think they don’t make sense, I’d recommend exploring that further and publishing a takedown of the economic way of thinking. I am not being facetious. If you can write a convincing critique, that would be worth sharing with everyone, and I’d be interested in reading it.
KevinDC
Mar 26 2021 at 8:11am
JFA is spot on. Yes, those are two distinct reasons for why something isn’t able to be used and made into a resource (in the economically relevant sense) but…whoever said there could only be one reason for it? I certainly didn’t – and I don’t think anyone else has either. Indeed, it’s trivially easy to come up with more and more examples of distinct reasons why this might happen. Maybe there isn’t a time or technology constraint – it could be a deficit in knowledge. Sometimes we simply stumble into finding out something can be usefully used with our current technology, and we just didn’t realize it. In that case, the limit wasn’t time or technology, it was simple awareness, and what previously wasn’t a resource becomes a resource. This, too is a distinct reason – but so what? The fact that there are large number of reasons for why this phenomenon can occur strengthens the argument, it doesn’t undercut it.
Jon Murphy
Mar 26 2021 at 9:34am
They are. But that doesn’t undermine anything here.
Knut P. Heen
Mar 24 2021 at 10:50am
The point is that scarcity implies a finite supply. The supply cannot be finite if there is an infinite number of suppliers. It is a mathematical point. The definition of infinity is that it is boundless.
There is a tendency for economists to forget that they are doing economics when they start doing the math. It is fine to use infinity arguments in mathematics. We should be more careful using it in economics.
Jon Murphy
Mar 24 2021 at 11:03am
Ok? I don’t understand your point here. Where are you going with all this.
Jon Murphy
Mar 24 2021 at 3:33pm
Knut-
I think you’re misunderstanding the purpose of the model. Of course infinite suppliers is impossible in real life. But that assumption is a modeling choice to make the math play nice. It’s not meant to be a description of reality. It’s meant to make the model tractable.
So yes, you are right that there is a difference between the mathematical and the real world. But Vernon is not claiming that there are infinite suppliers in the real world. The model is a useful way of understanding the real world.
In other words, his model is no more a paradox than a triangle is a paradox because, in reality, there are no shapes represented by infinitely thin lines that exist between three points.
KevinDC
Mar 24 2021 at 4:38pm
On a similar vein –
Understanding geometry is a very useful skill when playing pool. It helps you figure out how to direct the cue ball where you want it to go and to move the other pool balls where you want them. (Assuming you have better manual dexterity than my hopelessly clumsy self.)
One could object that the geometric modeling used in pool is “unrealistic.” And it is unrealistic – it assumes the pool ball is a perfectly round sphere, the table is a two dimensional, perfectly level frictionless surface, there is no air resistance, etc. Obviously none of these are true, but we act as if they are true because they make the geometry play nice, and provide useful information on how to achieve your goals. Similarly, economic models might make weird seeming assumptions like “infinite suppliers” or whatever to make the math play nice, but the models still have a great deal of predictive and explanatory power – which is the point!
Knut P. Heen
Mar 26 2021 at 9:46am
Suppose someone says that we need some kind of public project to enhance our society. Suppose further that the number of tax payers is infinite. Then the conclusion is that the project is “free” because an infinite number of people get together and pay a finite amount (zero per tax payer). This is essentially what the Arrow-Lind Theorem says.
A large finite number (like 8 billion) and infinite is not the same thing.
Jon Murphy
Mar 26 2021 at 10:28am
Right, but note the two things you changed:
First: You switched from a model to a policy. A positive model (ie a model that explains what is) is different from a normative model (ie a model that explains what ought to be). They are often similar on the surface, but are factually two very different things. In the positive model, simplifying assumptions do not matter as much. In the normative, they do.
Take, for example, the optimal tariff model. If we witness that Country A imposes some tariff on Country B and the net welfare of A increases. We can use the optimal tariff model to explain that result and the simplifying assumptions (such as the other country not retaliating, no public choice issues domestically, etc) don’t really matter. However, if we then want to use the optimal tariff model to guide policy, those simplifying assumptions really start to matter.
To bring this back, the fact that Vernon and Sabiou’s model has suppliers “increasing without bounds” as a means of positively explaining price formation does not matter a whole lot. If we were then going to take this model and make normative claims about what prices ought to be, then yes that assumption does indeed matter.
Second, you mistook how they use “infinite.” The number of firms is increasing without bounds; in other words, the number can keep growing as market conditions warrant. It’s not that the number of firms is infinity. It’s that they are increasing without bounds: infinite.
Justin
Mar 24 2021 at 12:58pm
The note is a little short to understand all the details from my perspective, but I don’t think he’s increasing the number of suppliers while holding the number of consumers constant. In that case you’d have a problem. It seems to me is saying there is a fixed number n of agents going to a market to trade and we take a limit as n goes to infinity. Scarcity comes from the number of consumers increasing in proportion with the sellers. You could say that this doesn’t make sense with an infinite numbers of agents, but that’s just a misunderstanding of how limits work. The limit as n goes to infinity is not the same as letting n equal infinity.
Vernon Smith
Apr 9 2021 at 6:10pm
The distribution of Min wta is not specified. So the question has no answer.
Suppose it is an infinite spike at zero. Whatever the declining consumer wtp demand, if it reaches zero at Qw, this is the amount taken by all buyers, the price is zero. As Hayek notes which goods are scarce and which not, requires a market to determine. The English “scarce” is used to define price > 0.
Suppose the Min wta supply price is c > 0, so the distribution is a spike at c. Where declining demand reaches c >0 determines consumption. There is a large excess supply not taken. If there is a next period, only the successful sellers are motivated to return, and so on into the future. If demand should grow, the price rises vertically above above c, and sellers return to satisfy the greater consumption at price, c.
The math is clear, I believe, and English words describe it.
Mark Z
Mar 23 2021 at 5:08pm
Is Shannan supposed to be Shannon?
Vernon smith
Apr 9 2021 at 7:55pm
Yes, thanks; bad vision!
kjetil haugen
Mar 24 2021 at 9:01am
Knut P. forgot this one:
https://reunido.uniovi.es/index.php/EBL/article/view/14821
Comments are closed.