The Demand and Supply of Public Goods
By James M. Buchanan
First Pub. Date
1968
Publisher
Indianapolis, IN: Liberty Fund, Inc.
Pub. Date
1999
Comments
First published in 1968 by Rand McNally & Company. Foreword by Geoffrey Brennan.
Copyright
The text of this edition is copyright ©: 1999 Liberty Fund, Inc. Picture of James M. Buchanan: File photo detail, courtesy Liberty Fund, Inc. James M. Buchanan, Charlottesville, Virginia, 1964.
- Foreword
- Ch. 1, A Methodological Introduction
- Ch. 2, Simple Exchange in a World of Equals
- Ch. 3, Simple Exchange in a World of Unequals
- Ch. 4, Pure and Impure Public Goods
- Ch. 5, Many Private Goods, Many Persons
- Ch. 7, The Publicness of Political Decisions
- Ch. 8, The Institutions of Fiscal Choice
- Ch. 9, Which Goods Should Be Public
- Ch. 10, Toward a Positive Theory of Public Finance
- Supplementary Reading Materials
 Pure and Impure Public Goods
Pure and Impure Public Goods
We have come part of the way in generalizing the models of simple exchange with which the analysis commenced in Chapter 2. The restrictive assumptions as to the identity of our two traders in both tastes and in productive capacity have been abandoned. Income effects have been introduced into the analysis. In this chapter, we propose to drop another one of the initial assumptions, that which requires purity in the public good. For the present, we shall remain in the two-person world. The limitation to two goods at the production level will be retained, although the introduction of impurity leads necessarily to a third
consumption good. We shall explore the process through which equilibrium is attained when one good is something less than wholly or purely collective in the strict sense.
By the orthodox definition a pure public good or service is
equally available to all members of the relevant community. A single unit of the good, as produced, provides a multiplicity of consumption units, all of which are somehow identical. Once produced, it will not be efficient to exclude any person from the enjoyment (positive or negative) of its availability. To use the terminology preferred by R. A. Musgrave, the principle of exclusion characteristic of goods produced in the market breaks down here. Nonexclusion applies in the extreme or polar sense. Additional consumers may be added at zero marginal cost.
This definition is highly restrictive, and it is not surprising that the modern theory of public goods has been criticized on this basis. Strictly speaking, no good or service fits the extreme or polar definition in any genuinely descriptive sense. In real-world fiscal systems, those goods and services that are financed publicly always exhibit less than such pure publicness. The standard examples such as national defense come reasonably close to descriptive purity, but even here careful consideration normally dictates some relaxation of the strict polar assumption. It is evident that the whole theory would be severely limited if it were to stand or fall on the correspondence of this purity assumption with observations from the real world.
Fortunately the theory has a much wider base, and I shall demonstrate that it retains general validity independent of the descriptive characteristics of particular goods and services. In so doing, however, I shall also show that attempts to employ the classification as a tool in determining what goods and services should be organized collectively rather than privately must be abandoned, at least provisionally. The theory of public goods when properly interpreted becomes applicable to
any good or service, quite independent of its physical attributes. The theory’s relevance depends upon the institutional arrangements through which the political group organizes the supply of goods and services. In one sense, the approach here amounts to an inversion of the theory as conceived by some modern scholars. Instead of using the model to classify the appropriateness of alternative institutional arrangements, I shall demonstrate the model’s usefulness and general validity with respect to all goods and services that happen, for any reason, to be organized and supplied publicly.
Private Goods as Public Goods
Initially, let us take a good that under normal circumstances we know to be purely private. A unit that is produced corresponds to a unit consumed by only one person, and neither its production nor its consumption generates, positively or negatively, relevant external or spillover effects on persons other than the direct consumer. If we can show that the theory of public goods properly interpreted can be made applicable even for this sort of good, then it should become clear that we can utilize the same tools for a good or service that falls anywhere along the whole indivisibility spectrum.
For simple illustrative purposes, think of such a good as bread. Under normal circumstances, a unit of this good, defined in physical units produced or consumed per unit time, can be transformed into only one consumption unit. That is to say, only one person can enjoy directly the benefits of a loaf of bread in a single time period. It is physically impossible for you and me to eat the same loaf of bread. Even here, however, we can analyze the attainment of trading equilibrium with the tools provided by the theory of pure public goods. The critical step is to define the good properly. Generically, “bread” is privately divisible among separate consumers, and we cannot apply the theory of indivisible goods to the demand and the supply of “bread” as so defined. We may, however, define the “good” that we propose to analyze in such a manner that it does embody the necessary indivisibility characteristics. To do so, all that is required is that we define our commodity in terms of
identifiable units. In this example, define the good to be analyzed as “my bread.” There will then be as many separate “my breads” as there are persons, all within the single generically defined commodity group “bread.” But with this relatively simple definitional step, we can proceed to apply the theory without qualification.
Take one of these
n goods, say, “your bread.” Assume, for any reason, that the community of which you are a member has decided that this is to be supplied publicly. You are not allowed to produce, purchase or consume “your bread” until and unless you are able to secure the permission of other members of the group. Let us assume the existence of a Wicksellian unanimity rule for making community decisions. In this case, the characteristics of equilibrium are not difficult to define. Since the marginal evaluation of “your bread” is zero for all other persons and over all quantities, it will be unnecessary for you to engage in “trade” with them. Equilibrium is attained when your own marginal evaluation equals the marginal cost of production. This is, of course, the same equilibrium that the market process generates. Nevertheless, the identity of the standard theory of markets and the theory of public goods in this instance is worth emphasizing. Note that, using the latter, we can say that the summed marginal rates of substitution between the “public good” and some numeraire private good must equal marginal cost. This statement of the necessary marginal conditions of optimality holds without qualification. We are, in this example, merely adding a string of zeros to a single positive value in the summation process.
The Unit of Joint Supply
The necessity of treating each person’s consumption good separately is, of course, dictated by the objective of utilizing the tools provided by the theory of public goods. Once we have demonstrated the possibility of such an extension, there need be no such analysis for a genuinely private good since, by definition, the standard theory of private-goods exchange applies. Our interest here is not with this theory but with extending the theoretical apparatus developed in application to purely public goods to cover “impure” goods, those neither purely private nor purely public. This raises the question as to whether the conditions for equilibrium can be derived in some fashion that will not require
n separate statements, one for each person’s identifiable units of possession.
Once again, it is useful to recall the theory of joint supply. This will allow us to introduce a simplification. The necessary condition for equilibrium is that the summed marginal evaluations of the consumption components must be equal to the marginal cost of the production unit. Apply this condition to the purely public good. The production unit, or unit of joint supply, provides or embodies
n-consumption units, when
n is the number of persons in the group. Since there is only one production unit, however, the analysis can be limited to this single unit dimension on the cost side. The same analysis may be extended readily to purely private goods, however, provided only that we make the
same summation over persons on the cost side as we do on the demand side. The general condition necessary for optimality in all cases is that summed marginal evaluation equals summed marginal cost, with the units appropriately defined.
The Fixity of Proportions—Equal Shares
Marshall’s theory of joint supply commences with the assumption that the final products or product components are in fixed proportions. If variability in proportions is allowed, additional conditions must be derived and the analysis becomes more complex. As we noted earlier, with a public good the assumption of pure publicness guarantees that different consumers have available to them equal shares. This begs the issue, however, and suggests a further examination into the precise meaning of the terms “equal shares” or “equal availability.” What do we mean by saying that a publicly supplied good or service is “equally available” to all members of the community?
First of all, as already noted, this does not imply that the marginal evaluations placed on the good by the separate consumers are equal. In some of the literature of modern public-goods theory, equal availability seems to mean that each consumer has available for his use the
same quantity of consumption units. This gets us nowhere, however, until we can clarify the meaning of the “same quantity.” What does it suggest to say that Mr.
A has the
same quantity of public good or service
X available to him as does Mr.
B?
Let us once again take a simple illustration, fire protection. How do we go about measuring quantity of such a service? One procedure might be to define units of service flow in terms of the probability that destructive fire will damage property. If fire protection provided by the community to Mr.
A is sufficient to insure that on any given day there is only a .0005 probability that his property will suffer fire damage in excess of $100, we can say that more protection is provided than if this probability should be .0007. This manner of defining the quantity of service flows utilizes
homogeneous-quality consumption units. This is, of course, the standard way in which we measure quantities of privately supplied goods and services.
If this procedure is followed, however, the theory of public goods does not carry us very far, if indeed it carries us anywhere at all. There are, in reality, no purely public goods if equal availability is measured in such terms as these. At this point, it is useful to recall the earlier apparent digression where the theory of public goods was extended to apply to the purely private good, “your bread.” We said that the commodity, “your bread,” was equally available to all members of the community. In that formulation, we could not have possibly been defining equal availability in terms of similar quantities of homogeneous-quality consumption units. We must have been applying some measurement procedure different from that which economists apply to fully divisible private goods and services.
Again the theory of joint supply is helpful. To the extent that a good or service, as produced, satisfies more than one demand, we can measure quantity, not in homogeneous-quality consumption units, but in
production units. And there is nothing inherent in the jointness of supply,
per se, which suggests that different demanders need enjoy or have available to them homogeneous-quality units for final consumption. This point is, of course, made evident in Marshallian joint supply, where final consumption components may be demonstrably different in some physically descriptive sense (meat and hides). The point is less apparent, but equally valid, with reference to publicly supplied goods and services. In our fire protection example, suppose that a fire station is physically located nearer to Mr.
A‘s residence than to Mr.
B‘s. In terms of homogeneous-quality final consumption, these two persons do not enjoy the same quantity of fire protection. However, the services of the fire station, given its physical location, are equally available to both
A and
B, and, as joint consumers, they may be said to enjoy the same quantity of the public good, fire protection, so long as the latter is defined strictly in
production or supply units.
The differentiation in the physical quality and in the quantity of consumption goods and services supplied to separate persons will, of course, be reflected in the different marginal evaluations placed on the jointly supplied inputs. Hence, in our illustration, even if
A and
B should have identical utility functions and identical incomes,
B will place a lower marginal evaluation on the publicly supplied service of fire protection for the simple reason that, translated into units relevant for his own consumption, he enjoys a lower-quality and smaller-quantity product. It is because of this translation of differential service flows into differential marginal evaluations that difficulties arise in any attempt to separate genuine differences in tastes from differences in physical service flows.
The analysis here suggests that the theory of public goods can be meaningfully discussed only when the units are defined as “those which are jointly supplied” and when “equal availability” and, less correctly, “equal consumption” refer only to jointly supplied production units or inputs, which may and normally will embody widely divergent final consumption units, measured by ordinary quality and quantity standards. Interpreted in this way, the theory becomes very general.
If a good or service is supplied jointly to several demanders or consumers, the question arises whether the “mix” among the separate components is fixed or variable. In Marshall’s example, the unit of production (the steer, the physical characteristics of which were initially assumed to be invariant) determined uniquely the meat and hides content in each jointly supplied bundle. In our own illustration, the
fixed location of the fire station determines uniquely the relative quality-quantity of the services received by
A and by
B. For any publicly supplied good or service, the availability of which is open to all members of a group, the proportions in the mix are set by the locational-technological characteristics of the supplied units.
*6 Once these are set, the analogue to the Marshallian fixed-proportion model is complete.
In the sense noted here, public goods or services will normally be multidimensional. Not only must the location of the fire station in the municipality be fixed, but all the other characteristics of the public service must also be specified to the extent that these influence in any way the quality-quantity of final consumption components received by different demanders-users. To simplify, we may use “location” as a surrogate for all such characteristics. In our fire station illustration, this amounts to assuming that the sole characteristic of the fire station that influences the relative quality-quantity of fire protection received by
A and
B is its location. It is evident, of course, that many such problems of dimensionality arise in the provision of almost any public good or service. A police force better trained to break up street riots than to track down safecrackers will nevertheless be equally available to citizens who have plate glass windows in main streets and to citizens who keep large cash sums in safes. But the relative amount of protection actually received by each group will depend on the technical mix of this composite force, this being the unit of joint supply in the appropriate jurisdiction. Once the technical characteristics of this unit are set, the physical consumption flows to the different demanders are combined in fixed proportions and the analogy with Marshall’s fixity in proportions is direct. If these characteristics are assumed to be determined by noneconomic, engineering considerations that are divorced from the respective preferences of the demanders, the theory of public goods can be applied without difficulty and emendation. No problem of determining the optimal mix among components in the jointly supplied unit need arise.
The Component Mix—the Technology of Public Goods
As the illustrative examples make clear, in ordinary cases of public-goods supply no such noneconomic considerations are paramount. The components in the appropriate units of joint supply can normally be varied within rather wide limits. Even if this should not prove possible in each instance, the theory should be generalized if at all possible to allow for such variability. It should be possible to lay down necessary conditions for optimality in the mix. The structure will remain seriously incomplete unless we can isolate, at least conceptually, the forces that make for distinct variations in the mix among the consumption components in a jointly supplied public good. Under what conditions should the fire station be located near
A rather than
B? Under what conditions should the police force be trained primarily to break up street riots rather than to locate burglars? We need to examine the conditions for equilibrium or optimality in the component mix in addition to the more familiar conditions for equilibrium or optimality in the quantity of the production units that are to be supplied.
Note that this problem arises only with publicly supported goods and services that are impure. They must be neither wholly private, in the sense of no spillover benefits or harms arising from their production or consumption, nor wholly public, in the sense of strictly equal consumption of homogeneous-quality units of good or service. In the first case, even if the supply should be publicly organized, there is no question of defining the optimal mix since each demander’s preferences can be satisfied independently and separately. In the second case, there will be no interpersonal quality-quantity variability by definition. The interesting cases are those falling between these polar limits. And here interpersonal and intergroup variability can readily be incorporated into the production process, even within the overall technological constraints that dictate the relative efficiency of joint supply. In illustrative terms, the fire station can readily be located at any one of several places, each one of which embodies a different mix among consumption components, despite the fact that, wherever located, within wide limits,
A and
B will still find it relatively more efficient to secure their fire protection services jointly rather than separately.
Equilibrium in the Mix
Let us now return to our simple Tizio-Caio model to discuss this problem concerning optimality in the mix, one that has not been adequately developed in the modern literature. In the model of simple exchange, introduced first in Chapter 2, we assumed that one of the two goods was purely public in the strictest definitional sense. We presumed, without really raising the issue for serious critical scrutiny, that each of the two consumers enjoyed equal quantities of homogeneous consumption units. That is to say, we assumed that the killing of one mosquito, whenever or wherever, provided an equal quality service flow to Tizio and to Caio. As the discussion in the preceding sections suggests, this highly restrictive feature of the model must now be modified. We propose to make the two consumption components enjoyed by Tizio and Caio into two conceptually distinct goods. Both Tizio and Caio place positive valuation on mosquito repelling services, but let us assume that the two men sleep at different locations. Therefore, the location of the public good or service can modify the mix between the two components.
If it should be technologically necessary to release mosquito repellent at only one place, the earlier analysis would not be affected in any way and no additional conditions need be derived. The fact that, in some descriptive sense, the final consumption components should amount to quite different goods would in this case be wholly irrelevant to the analytics. As we have suggested this seems an overly restrictive model, and we want to examine one in which the mix is variable. Assume that although Tizio and Caio will always find it relatively efficient to control mosquitoes jointly rather than separately, variations are possible in this production-supply process that within wide limits will favor one or the other of the two components. We want to examine the process through which Tizio and Caio attain some equilibrium supply of mosquito repellent, but, also, we want to examine the process through which they attain some equilibrium mix among consumption components that characterize this public good. How much repellent or repellent services should be produced, and where should this activity take place?
The Mix of a Pure Public Good: The Limiting Case of One-for-One Correspondence Among Consumption Components
The problem of determining the optimal mix among consumption components in a jointly supplied production unit when this mix is variable may be discussed with the geometrical constructions to be introduced in this section. It will be helpful to present this construction first under the assumption that the mix is completely invariant in an extreme or limiting case where there is a one-for-one correspondence among the separate consumption components. Let us say that technological characteristics are such that every person receives equal quantities of homogeneous-quality consumption units from each unit of public good that is produced.
 |
| Figure 4.1 |
In Figure 4.1, this case becomes easy to diagram. The final consumption components enjoyed by the two demanders, Tizio and Caio, are measured along the abscissa and ordinate, respectively. Production can take place only along the 45° line as shown. A unit of production becomes two units of consumption. A unit of final consumption supplied to one person automatically insures that a unit is also supplied at the same time to the remaining consumer, or consumers, in the group. It becomes impossible, by definition, to produce a unit of
y
t, the consumption component enjoyed by Tizio, without at the same time, and jointly, producing precisely one unit of
y
c, the consumption component enjoyed by Caio. As the geometrical construction suggests, the only problem in this highly restricted model is one of determining the optimal extension of production along the 45° ray. No problem of determining the optimal or equilibrium mix arises here.
The construction is useful, even in such a highly restricted model, in indicating that the separate consumption components need not be physically or descriptively identical if consumption units are defined only in terms of the contents of the production units. Tizio may be receiving mosquito repellent and Caio tick repellent, to vary our illustration, while the production of insect repellent qualifies as that of the pure public good. All that is required here is that there be a one-for-one correspondence among the separate consumption components in the mix and that this mix be invariant. In terms of
production units, all demanders are receiving or enjoying identical goods here.
Consumption units enjoyed by the separate parties may be (although they need not be) quite different one from the other in a descriptive sense.
The Mix of a Pure Public Good: Fixed Proportions
There need not exist such a one-for-one correspondence among separate consumption components in all public goods, even in those which can be classified as “purely public” in some more general sense. If units of final consumption enjoyed by each demander are measured
independently in some physical dimension the quantities received by each person need not match up one-for-one. Consider once again fire protection, received by Tizio and Caio from a fixed-location fire station that is not equidistant from their properties. Each expansion in the production of the gross commodity, fire protection, at this fixed location will provide additional protection to both persons. But this need not be one-for-one. If, for instance, the fire house is nearer to Tizio than to Caio, an additional set of hoses on the fire engine may add three times the quantity of protection to Tizio that it adds to Caio. Production here can take place only along the ray
h on Figure 4.1, indicating a three-for-one, not a one-for-one ratio. Note that here, as before, the pure public good is equally available to both demanders in
production unit terms.
It would, of course, always be possible to redefine quantity units of consumption in such a way as to restore the one-for-one correspondence. If each consumption unit is measured in units of quantity contained in each
unit of production, then each person enjoys equal quantities, by construction. It seems probable that this procedure has been implicit in much of the discussion of the theory, which has not included discussion of the mix among components. This convention of redefining quantity units may be helpful in certain cases, but here it obscures the very problem that we seek to examine. Once it is fully recognized that, in terms of final consumption units enjoyed, equal availability means little or nothing, the question that arises concerns the possibility of varying the component mix.
The Mix Under Variability of Proportions
Any general model must allow for variability in the mix among separate consumption components of jointly supplied goods and services, whether or not these be publicly provided. The two preceding models, in which such variability is not allowed, serve only to emphasize the restrictiveness of the standard public-goods assumption. It is difficult to think of practical public-goods examples where variability, within some limits, is not feasible. Mosquito repellent can be released in many parts of the island; fire stations can be located in many places; police forces can be variously trained.
Once this sort of variability is allowed, however, the necessary conditions for optimality in this mix must be determined in addition to the necessary conditions for optimality in the extension of production of the public good or service. Public-goods theory, as developed over the last quarter-century, has been almost exclusively devoted to the second of these problems, as has been almost all of the discussion in Chapters 2 and 3 above.
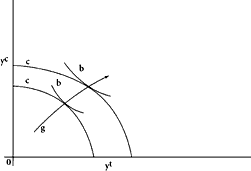 |
| Figure 4.2 |
The analysis for the two-person, two-component model can be presented geometrically. In Figure 4.2, as in Figure 4.1, the two consumption components are measured along the axes. One simplifying assumption is necessary at the outset. The total cost function for each component, when and if
separate production takes place, is linear. This assumption insures that if there are no efficiencies in joint production, iso-cost curves will also be linear. The impure public good that we want to analyze does, however, embody net efficiency in joint production of the two components. This efficiency is indicated by the convexity of the iso-cost curves, the
c curves in Figure 4.2.
As these curves are drawn, note that individual behavior under independent production would not generate external economies. If each person should be required to produce his consumption component separately for his own use, it will be efficient for him to exclude the other person from the enjoyment of any spillover benefits. An alternative construction could be introduced (in which the
c curves exhibit positive slopes over some ranges, as do those in Figure 4.4) which would incorporate observed external economies under wholly independent behavior. In this construction, joint production would remain efficient, but, also, nonexclusion would characterize privately organized supply. For analytical purposes at this point, either of these two constructions is suitable. All that we require is that the joint supply of the two components be relatively more efficient than separate supply.
The iso-cost curves are derived by mapping onto the surface of Figure 4.2 the contour lines from the appropriate total cost surface. Cost is measured in units of some numeraire private good, along an axis extended outward from the surface of the figure.
*7 These iso-cost contours indicate the marginal rate of substitution between the two consumption components on the production side. Before the necessary conditions for optimality in the mix between components can be derived, we need to determine, for each level of production, the rates at which these components may be substituted, one for the other, in the combined evaluation of the two traders. To simplify the presentation here, we have assumed that Tizio and Caio are interested solely in the consumption services that they receive directly. That is to say, neither person places a value on consumption flows to the other person. For a single person, therefore, indifference contours mapped onto Figure 4.2 would take the form of a series of parallel lines vertical to his own service flow axis. We are interested, however, in the joint or combined evaluation that the two men place on the two components in the mix. To secure a total benefit or total evaluation surface it is necessary to add the two individual benefit or evaluation surfaces in the private-goods or numeraire dimension. Once this step is taken, we can draw contour lines which can be mapped onto Figure 4.2 as iso-benefit or iso-evaluation curves. One such set is shown as the
b curves. So long as diminishing marginal rates of substitution between the consumption component and money hold for each person, the iso-benefit curves must exhibit the convexity properties shown by the
b curves.
The tangency between an iso-outlay and an iso-benefit curve is a necessary marginal condition for optimality in the mix of the two components at each level of production. The path along which production should proceed is indicated, therefore, by the locus of such tangency points, the ray labeled
g in Figure 4.2. In this construction, we have again neglected income-effect feedbacks. Full incorporation of these would have made it impossible to derive iso-evaluation contours independent of the cost-sharing arrangements over inframarginal ranges, and these effects might also have modified the shape of the optimal-mix path over these ranges.
 |
| Figure 4.3 |
Once the ray or path of optimal mix among separate components in the jointly supplied unit of production is determined, there remains only the determination of the rate of production along this ray or path. The solution here is quite straightforward, and it is the familiar one. It is represented by taking the derivative of the cost function along this optimal-mix path and equating it with the derivative for the total benefit function taken along the same path. Figure 4.3, which has a familiar look about it to economists, depicts this solution geometrically. Measured along the abscissa are units of production along the defined path. Measured along the ordinate are units of the private or numeraire good. The necessary conditions for optimal extension in production are satisfied when the slopes of the two functions are equal, again recalling the required neglect of income-effect feedbacks for this simplified construction here.
Note that this statement of the necessary marginal conditions is equivalent to that presented earlier in the simpler models. At the margin, a unit of production embodies two component “goods.” In one sense, therefore, the marginal cost of supplying this combination represents the summed marginal costs of the two components. On the other side of the equation, the marginal benefits placed on the two components must equal the summation of the evaluations of the two demanders.
With this extension of the basic theory to the impure good which embodies widely varying proportions of the several components, but which is still characterized by efficiencies in joint supply, the analysis moves significantly toward generality. Although the construction becomes complex, the analysis is not modified in its essentials when we allow the separate demanders to place positive or negative evaluations on components in the mix other than the service flows which they receive directly. The owner of the plate glass window who is fearful of street riots can be allowed to place some value on the tracking down of safecrackers in the neighborhood, the prime interest of his neighbor. The characteristics of equilibrium are not modified. Both the purely public good and the purely private good become special cases of the more general theory that emerges here.
As we have noted, the separate demanders may value wholly different or quite similar components in the unit of jointly supplied good. For many public services, national parks for example, we normally think of separate persons enjoying similar physical facilities. Nevertheless, even such services as this can be best interpreted as embodying separate components. Where should a new park be constructed, and which existing ones should be extended? The decision on such matters, insofar as efficiency criteria dictate, is precisely equivalent to that of determining the optimal mix among components. A decision to expand park facilities in Nevada rather than in West Virginia is a choice of a mix that includes a relatively smaller proportion of consumption units benefiting an easterner, and a relatively larger proportion of the units benefiting a westerner.
External Economies
in Consumption: “Publicness” Without Orthodox Economies of Joint Supply
The phenomenon of joint supply has been the central feature of all public goods and services in the analysis developed to this point. The bases upon which individuals are motivated to organize the joint supply of any particular good or service has not been explored in detail, but implicit in the above discussion and in much of the standard literature is the assumption that technical characteristics inherent in the production process serve to make common sharing relatively efficient. The external economies arise in production, not consumption. Consider the classic examples. Why do the separate fishermen on the island refrain from building separate lighthouses? The act of producing a single lighthouse provides spillover or external benefits to all fishermen. Externally benefited parties care not at all whether or not the producer himself
consumes the services that he produces. We propose to consider in this section the quite different model in which the external economies arise from the
act of consuming. In this model, there need be no external economies from production in the orthodox sense, hence, no jointness efficiencies. With some stretching of the analysis, this model can be incorporated into the general public-goods model already developed.
Earlier in this chapter, the possible extension of the basic analytical model to purely private goods and services was examined, primarily for purposes of illustrating the generality of the tools. This discussion was then followed by showing how “impure” public goods may be brought into the analysis. Impurity or imperfect publicness in this respect was defined, however, as any departure from the availability of “equal quantities of homogeneous-quality consumption units” to all customers. Despite the presence of such impurities, the public-goods model was shown to hold so long as joint supply collectively or cooperatively organized is present.
We now want to assume away all jointness in supply, at least in this standard sense. We want to examine those instances where the external economies that may be present arise solely from the act of consumption. There is here, by definition, no spillover from production as such.
Consider a modified Tizio-Caio example. Through some daily expenditure of effort in digging out a special root and eating it, a person can make himself temporarily immune from a highly communicable disease. What form do the externalities take in this example? Tizio is not affected by Caio’s
production of the immunizing agent; there are no economies of joint production by definition. Tizio is, however, affected by, and hence interested in, Caio’s
consumption of the immunizing agent since Caio’s immunity protects Tizio also and
vice versa. Only in
consumption is a “public good” produced.
A familiar real-world example that closely approximates this case arises in educational services. There are few, if any, necessary economies of joint supply on a scale sufficiently large to warrant consideration of collective organization. It is widely acknowledged, however, that important external economies or spillovers are generated in the act of consuming educational services. As a member of the political community, say a municipality, you are interested in the utilization or consumption of educational services by the child that lives in that community.
The extension of our basic theory to cover this case is not difficult. Here we resort to the approach already suggested when we treated any purely private good as a public good.
Each person’s consumption or utilization of the service must be considered separately, as an independent public good. You, as a member of the community, are interested here in
n separate public goods,
each one representing the educational services actually consumed by a single child in the same jurisdiction. For each of these
n goods or services, joint supply in the orthodox fashion holds, and the necessary condition for full equilibrium may be derived as before. The marginal rates of substitution summed over all individuals in the group must be equal to the marginal cost of producing the service. When we discussed treating a purely private good as public, the procedure amounted to adding a series of zeroes to a single positive value. In the present case, where the external economies arise in consumption, we are confronted with an impure or in-between situation. Normally, the actual consumer of the services will place some differentially higher value on this consumption than his fellows. Such goods and services tend to exhibit considerable divisibility. In the case of educational services, a significantly higher evaluation will be placed on the services by the direct beneficiary, the family of the child who consumes. To this higher evaluation will normally be added, not a string of zeroes, and not a string of equal values, but a whole series of lower but still positive values.
Note that through this device of considering each person’s consumption as a separate public service, we have converted the model into one where joint supply necessarily applies. Inherent in the education of the single child in the community is the joint supply of “this child’s education” to all other members of the relevant group. The demands of all members are jointly met in the consumption of education by the single child. The analytical model developed earlier for other cases of impure public goods now holds without qualification. The problem of determining the optimal mix now becomes one of locating the quality standards that should characterize the educational services to be supplied to the particular child.
 |
| Figure 4.4 |
For simplicity in illustrating this point, let us resort to a two-person model again, with some variations, and remaining within the educational services illustration. Let us take Family Brown as our direct consumer. It has one child of school age, Charlie Brown, and the family, as a decision unit, is directly interested in Charlie’s consumption of educational services. The rest of the community we treat here as a single person, called
ROC, and this unit is also interested in the consumption of education by Charlie Brown. In Figure 4.4, we illustrate the problem as before by indicating possible variations in the mix among separate components.
We must define the units along the two axes in Figure 4.4 with some care. Along the horizontal axis, we measure physical service flows to the direct beneficiary of the child’s utilization of educational facilities: in our case, Family Brown. Along the vertical axis, we measure physical service flows to the spillover beneficiaries stemming from the same utilization of educational facilities by the same child. Conceptually, these service flows are objectively computable. For purposes of analysis here, we may consider them to be measured in terms of reduced probabilities that the child will, when he becomes an adult, impose direct costs on the beneficiary. Such costs might take any of several forms: criminal, delinquent or antisocial behavior; substandard contribution to collectively organized activities; corrupt or suspect behavior in political process. The point to be emphasized is that the consumption of education by a single child generates some such physical flow of services both to the direct beneficiaries and to spillover beneficiaries. These physical flows are measured on the axes of Figure 4.4. They must be kept conceptually distinct from individuals’
evaluations placed on these flows. At this point, we are not directly concerned with the values, positive or negative, that direct or indirect beneficiaries may place on such service flows. Nor are we concerned here with problems of measuring such physical service flows in any empirical sense. Errors in estimation may, of course, cause individuals to place negative evaluations on service flows that objectively generate positive values. And, contrariwise, individuals may place positive evaluations on wholly imaginary flows of services.
As suggested, the behavior of direct beneficiaries in generating the consumption of educational facilities by a single child will normally provide some flow of services to other members of the community. Nonexclusion tends to be characteristic of such externalities. The privately generated behavior of the direct beneficiary, the family of the child who is being educated, may be depicted by its shift along the path
g in Figure 4.4. As our earlier analysis of the public-goods mix suggested, if there is only one sort of education that can be consumed or utilized by the child, this path is unique. The incorporation of the interests of spillover beneficiaries, through some collectivization process, will serve only to shift the position of equilibrium outwards along the path
g, say, from
P to
P”.
It seems obvious from the example here, however, that such “fixity in proportions” is not likely to occur. There are many variables in the education mix, and the “bundle” of facilities actually utilized by the child may vary within rather wide limits. It also seems reasonable that some of this variability can be related rather directly to the relationships between direct and indirect beneficiary service flows, the units measured along the axes in Figure 4.4. The education bundle can surely be modified to shift somewhat the proportions between the two categories of service flows. Own-family benefits may stem primarily from educational inputs that generate higher income expectations for the child, while spillover benefits may stem primarily from educational inputs that generate higher “cultural or citizenship” expectations. As surrogates for these two variables, we may think of vocational or professional versus general or classical education.
If such variability is possible, the optimal mix among components will be determined in the same manner that we have presented with respect to the more orthodox impure public good. Some generalizations may, however, be made here, suggesting that the analysis is not wholly without relevance or applicability to real-world problems. Consider the problem of determining the necessary conditions for optimality in the education of a single poor child as compared with the same conditions in the education of a single rich child. Presumably, the evaluation placed on the direct service flows to the own-family will be less in the former case than in the latter, hence the proportion of costs borne by the
ROC will be greater. This suggests that, optimally, the education of the relatively poor child, or the child from poor parents, should contain a larger element of general material than that of the relatively rich child. Such generalizations from the analysis must, of course, be made with great care and with many qualifications. The direction of emphasis in variability may not be that which has been suggested here at all; also, efficiency considerations alone may not be of decisive importance. The implication is only that, if properly developed, the conceptual analysis here can lead to certain limited real-world predictions.
It must again be emphasized that, in treating of external economies that arise in the activity of consuming itself, each person’s or family’s activity must be considered as a separate public service in order to bring the analysis within the orthodox framework. One cannot combine the
n separate “goods” into “education of all children” and employ the standard analysis. If this mistake is made, basic misunderstanding of this whole category is likely to arise. When we try to consider several persons’ consumption or utilization of services simultaneously, we are really combining several separate externality relationships, with many resulting difficulties.
This analysis has important implications for the institutional arrangements of such consumption activities. What the analysis, along with the example, suggests is that the attainment of full equilibrium may involve participation of the whole membership of the community in financing the consumption activity of the single person, in the extreme cases, each person in the group, taken separately. What the analysis does not suggest is that the consumption activities of all persons, in our example, for all children, be jointly organized and supplied. Economies in the joint production of services for several persons may arise, of course. But such production economies are over and above, and quite different from, those consumption externalities that we have considered here. It is the latter which provide the basic motivation for potential collective-cooperative organization. Institutionally, the provision of facilities allowing the relevant consumption activity may be privately organized. Education may be supplied by private firms if this should prove the most efficient arrangement. The rest of the community may join with the direct beneficiary, the family, in purchasing privately supplied educational facilities. Equilibrium may well be attained most efficiently through ordinary competitive organization of the actual facilities, provided only that the community act somehow as a partner in the purchasing process. The incentive for cooperative action in such cases stems from the spillover benefits of consumption as such.
This case may again be contrasted with the orthodox public-good case when the spillovers or externalities arise from jointness and nonexcludability on the production side. Contrast education and police protection in this respect. You join forces with your neighbors in the municipality to finance education because you secure some benefit, for which you are willing to pay, from the consumption of services by your neighbor’s child. You are willing to join forces with these same neighbors to produce, directly or indirectly, police protection (for both yourself and your neighbor) not because you are specifically interested in their own lives and property being protected, but because through joint action you can secure protection of your own life and property more efficiently. To bring the first case into the strict confines of the model developed to apply to the second case, which is basically the model for joint supply, we have shown that it is necessary to consider each person’s separate consumption as an independent good. Because there is required here the organization of
n separate goods, there is no apparent argument for monolithic supply. The direct implication for institutional structures is clear; with production externalities there is a particular efficiency reason for considering publicly managed or controlled supply of service facilities. With consumption externalities, the type of organization should be determined strictly by more orthodox efficiency criteria. The argument for “public schools” (as opposed to “public financing of education”) must rest on a different footing from the argument for “public police protection.”
A Formal Summary
We may summarize the extensions of the analysis introduced in this chapter by reference to the algebraic statements for equilibrium that were first presented in Chapter 2. Let us return to the Tizio-Caio model employed in that chapter for simplicity in exposition. Following the statements of conditions (9) and (10) in that chapter, we said: “… the conditions are fully general for two-person, two-good exchange, and these same statements encompass any degree of externality or publicness in
x2.” If this earlier proposition holds, it should now be possible to summarize the analysis of Chapter 4 adequately through resort to these very general conditions for public-goods equilibrium. Conditions (9) and (10) are reproduced below for convenience. Recall that the superscripts refer to individuals;
x1 is the private good,
x2 the public good.
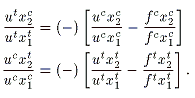 |
(9) (10) |
The
u‘s represent partial derivatives of the utility functions, the
f ‘s partial derivatives of the cost functions facing the two persons. In more familiar terminology, the left-hand side of (9) represents Tizio’s marginal evaluation of Caio’s activity of producing the good,
x2, for his (Caio’s) own consumption. The first term in the bracket represents Caio’s own marginal evaluation of this same activity, while the second term represents his marginal cost. Under fully independent behavior, the bracketed terms sum to zero. The terms in (10) are similarly explained, with only the position of the two persons reversed.
Let us now consider four possible cases: (1) the pure private good, (2) the pure public good, (3) the impure public good characterized by indivisibilities, (4) the good that exhibits external economies in consumption but not in production.
The first case is straightforward and need not be examined in detail. Tizio will place no marginal evaluation on the production-consumption of
x2 by Caio, and Caio will not positively value similar activity by Tizio. The left-hand terms in both (9) and (10) become zero, and the conditions reduce to the familiar statements for equilibrium under wholly private adjustment.
The second case is also simple. Here we may take the first term out of the bracket and shift it to the left-hand side of the equation, producing the more familiar summation of marginal evaluations over the two individuals which is then equated to the marginal cost of supplying the good. In this case, we may drop either one of the two equations, (9) or (10), since they make identical statements. Each person’s evaluation of the production-consumption activity of the other is fully equivalent to his evaluation of his own activity.
The third case is somewhat more difficult. Here the same quantities of homogeneous-quality consumption units are not available to both demanders, so that, even on the assumption of identical tastes, the evaluation that Tizio places on his own activity differs from that which he places on Caio’s activity. The same relationship holds for Caio. Note that this case covers both the fixed proportion and the variable proportion good, since the conditions (9) and (10) do not relate to the definition of optimality in the component mix. Because the externalities here arise solely from production, from the relative efficiency of joint supply, either (9) or (10) may be dropped since production will tend to take place at only one “location.” This case is different from the second, however, in that (9) and (10) will no longer be identical. Here either technological considerations will determine the precise location of
x2 or, more generally, the optimal mix will be determined by a consideration of both evaluation and cost factors. Before (9) or (10) is satisfied, these subsidiary conditions defining optimality in the component mix must be fulfilled.
In the fourth case, it is impossible to drop one of the two statements. Here the externalities arise not from production or joint-supply indivisibilities but from consumption activity, as such. Two separate collective or public goods must be considered,
x
t2 and
x
c2, the first being the consumption of
x2 by Tizio, the second being the consumption of
x2 by Caio. For each of these two quite separate goods, the familiar public-goods conditions hold, and for each, the subsidiary conditions as to optimal mix must also be added. In this case, conditions (9) and (10) say quite different things, the one relating to one public good, the other to another.
Bibliographical Appendix
The initial criticisms of Samuelson’s formulation of the theory of public goods were largely based on the limited applicability of the polar model [see Julius Margolis, “A Comment on the Pure Theory of Public Expenditure,”
Review of Economics and Statistics, XXXVII (November 1955), 347-49; G. Colm, “Comments on Samuelson’s Theory of Public Finance,”
Review of Economics and Statistics, XXXVIII (November 1956), 408-12]. In his treatise, R. A. Musgrave recognizes the limitation of the full-exclusion model. This recognition was, perhaps, instrumental in his development of the category of “merit goods” [
The Theory of Public Finance (New York: McGraw-Hill, 1959), Ch. 1]. This Musgrave category has been carefully examined by J. G. Head [“On Merit Goods,”
Finanzarchiv 25 (March 1966), 1-29]. In his 1966 paper, Musgrave analyzed several cases [“Provision for Social Goods” (Mimeographed, September 1966)]. In his second and third papers, and also in his later comment, Paul A. Samuelson responded to the criticisms concerning the polarity of his model [“Diagrammatic Exposition of a Theory of Public Expenditure,”
Review of Economics and Statistics, XXXVII (November 1955), 350-56; “Aspects of Public Expenditure Theories,”
Review of Economics and Statistics, XL (November 1958), 332-38; “Public Goods and Subscription TV: Correction of the Record,”
Journal of Law and Economics, VII (October 1964), 81-84; “Pure Theory of Public Expenditure and Taxation” (Mimeographed, September 1966)].
In my own review of Musgrave’s treatise, I suggested the relevance of a model that would include goods embodying varying degrees of “publicness,” based on a generalization of the external economies notion [“The Theory of Public Finance,”
Southern Economic Journal, XXVI (January 1960), 234-38]. Such a model was developed provisionally by Otto A. Davis and Andrew Whinston [“Some Foundations of Public Expenditure Theory” (Mimeographed, Carnegie Institute of Technology, November 1961)]. J. C. Weldon, in his comment on Breton’s paper, expressed the same objective and presented a different model [“Public Goods and Federalism,”
Canadian Journal of Economics and Political Science, XXXII (May 1966), 230-38].
The literature on external economies and diseconomies is, of course, exclusively devoted to analyzing “impure” goods and services. Several relatively recent contributions may be noted here [R. H. Coase, “The Problem of Social Cost,”
Journal of Law and Economics, III (October 1960), 1-44; Otto A. Davis and Andrew Whinston, “Externalities, Welfare, and the Theory of Games,”
Journal of Political Economy, LXX (June 1962), 241-62; James M. Buchanan and Wm. Craig Stubblebine, “Externality,”
Economica, XXIX (November 1962), 371-84; Ralph Turvey, “On Divergencies Between Social Cost and Private Cost,”
Economica, XXX (August 1963), 309-13; E. J. Mishan, “Reflections on Recent Developments in the Concept of External Effects,”
Canadian Journal of Economics and Political Science, XXXI (February 1965), 3-34; Charles Plott, “Externalities and Corrective Taxes,”
Economica, XXXIII (February 1965), 84-87; S. Wellisz, “On External Diseconomies and the Government-Assisted Invisible Hand,”
Economica, XXXI (November 1964), 345-62; Otto A. Davis and Andrew Whinston, “On Externalities, Information, and the Government-Assisted Invisible Hand,”
Economica, XXXIII (August 1966), 303-18; James M. Buchanan and Gordon Tullock, “Public and Private Interaction Under Reciprocal Externality,” in
The Public Economy of Urban Communities, edited by J. Margolis (Resources for the Future, 1965), pp. 52-72].
Specific problems that arise in the determination of the mix of an impure public good have been discussed by Carl Shoup and Douglas Dosser [Shoup, “Standards for Distributing a Free Governmental Service: Crime Prevention,”
Public Finance, XIX (1964), 383-94; Dosser, “Note on Carl S. Shoup’s ‘Standards for Distributing a Free Governmental Service: Crime Prevention,’ ”
Public Finance, ibid., 395-402].
Some aspects of specific consumption externality in education have been analyzed by Mark Pauly [“Mixed Public-Private Financing of Education: Efficiency and Feasibility,”
American Economic Review, LVII (March 1967), 120-30]. In a more general setting, some of these problems have been discussed by Burton Weisbrod [
External Benefits of Public Education (Princeton: Industrial Relations Section, Princeton University, 1964)].
Chapter 6

