The Purchasing Power of Money

CHAPTER II
PURCHASING POWER OF MONEY AS RELATED TO THE EQUATION OF EXCHANGE

§ 1

WE define money as what is generally acceptable in exchange for goods.*5 The facility with which it may thus be exchanged, or its general acceptability, is its distinguishing characteristic. The general acceptability may be reënforced by law, the money thus becoming what is known as "legal tender"; but such reënforcement is not essential. All that is necessary in order that any good may be money is that general acceptability attach to it. On the frontier, without any legal sanction, money is sometimes gold dust or gold nuggets. In the Colony of Virginia it was tobacco. Among the Indians in New England it was wampum. "In German New Guinea the bent tusks of a boar are used as money. In California red birds' heads have been used in the same way."*6 Stone money and shell money are so used in Melanesia.*7 "In Burmah Chinese gambling counters are used as money. Guttapercha tokens issued by street car companies in South America are said to be used in the same way."*8 Not many years ago in a town in New York state, similar tokens got into local circulation until their issue was forbidden by the United States government. In Mexico large cacao beans of relatively poor quality were used as money, and on the west coast of Africa little mats were used.*9 The list could be extended indefinitely. But whatever the substance of such a commodity, it is general exchangeability which makes it money.

On the other hand, even what is made legal tender may, by general usage, be deprived of its practical character as money. During the Civil War the government attempted to circulate fifty-dollar notes, bearing interest at 7.3 per cent, so that the interest amounted to the very easily computed amount of a cent a day. The notes, however, failed to circulate. In spite of the attempt to make their exchange easy, people preferred to keep them for the sake of the interest.*10 Money never bears interest except in the sense of creating convenience in the process of exchange. This convenience is the special service of money and offsets the apparent loss of interest involved in keeping it in one's pocket instead of investing.

There are various degrees of exchangeability which must be transcended before we arrive at real money. Of all kinds of goods, perhaps the least exchangeable is real estate. Only in case some person happens to be found who wants it, can a piece of real estate be traded. A mortgage on real estate is one degree more exchangeable. Yet even a mortgage is less exchangeable than a well-known and safe corporation security; and a corporation security is less exchangeable than a government bond. In fact persons not infrequently buy government bonds as merely temporary investments, intending to sell them again as soon as permanent investments yielding better interest are obtainable. One degree more exchangeable than a government bond is a bill of exchange; one degree more exchangeable than a bill of exchange is a sight draft; while a check is almost as exchangeable as money itself. Yet no one of these is really money for none of them is "generally acceptable."

If we confine our attention to present and normal conditions, and to those means of exchange which either are money or most nearly approximate it, we shall find that money itself belongs to a general class of property rights which we may call "currency" or "circulating media." Currency includes any type of property right which, whether generally acceptable or not, does actually, for its chief purpose and use, serve as a means of exchange.

Circulating media are of two chief classes: (1) money; (2) bank deposits, which will be treated fully in the next chapter. By means of checks, bank deposits serve as a means of payment in exchange for other goods. A check is the "certificate" or evidence of the transfer of bank deposits. It is acceptable to the payee only by his consent. It would not be generally accepted by strangers. Yet by checks, bank deposits even more than money do actually serve as a medium of exchange. Practically speaking, money and bank deposits subject to check are the only circulating media. If post-office orders and telegraphic transfer are to be included, they may be regarded as certificates of transfer of special deposits, the post office or telegraph company serving the purpose, for these special transactions, of a bank of deposit.

But while a bank deposit transferable by check is included as circulating media, it is not money. A bank note, on the other hand, is both circulating medium and money. Between these two lies the final line of distinction between what is money and what is not. True, the line is delicately drawn, especially when we come to such checks as cashier's checks or certified checks, for the latter are almost identical with bank notes. Each is a demand liability on a bank, and each confers on the holder the right to draw money. Yet while a note is generally acceptable in exchange, a check is specially acceptable only, i.e. only by the consent of the payee. Real money rights are what a payee accepts without question, because he is induced to do so either by "legal tender" laws or by a well-established custom.*11

Of real money there are two kinds: primary and fiduciary. Money is called "primary" if it is a commodity which has just as much value in some use other than money as it has in monetary use. Primary money has its full value independently of any other wealth. Fiduciary money, on the other hand, is money the value of which depends partly or wholly on the confidence that the owner can exchange it for other goods, e.g. for primary money at a bank or government office, or at any rate for discharge of debts or purchase of goods of merchants. The chief example of primary money is gold coin; the chief example of fiduciary money is bank notes. The qualities of primary money which make for exchangeability are numerous. The most important are portability, durability, and divisibility.*12 The chief quality of fiduciary money which makes it exchangeable is its redeemability in primary money, or else its imposed character of legal tender.

Bank notes and all other fiduciary money, as well as bank deposits, circulate by certificates often called "tokens." "Token coins" are included in this description. The value of these tokens, apart from the rights they convey, is small. Thus the value of a silver dollar, as wealth, is only about forty cents; that is all that the actual silver in it is worth. Its value as property, however, is one hundred cents; for its holder has a legal right to use it in paying a debt to that amount, and a customary right to so use it in payment for goods. Likewise, the property value of a fifty-cent piece, a quarter, a ten-cent piece, a five-cent piece, or a one-cent piece is considerably greater than its value as wealth. The value of a paper dollar as wealth—for instance, a silver certificate—is almost nothing. It is worth just its value as paper, and no more. But its value as property is a hundred cents, that is, the equivalent of one gold dollar. It represents to that extent a claim of the holder on the wealth of the community.

Figure 1 indicates the classification of all circulating media in the United States. It shows that the total amount of circulating media is about 8½ billions, of which about 7 billions are bank deposits subject to check, and 1½ billions, money; and that of this 1½ billions of money, 1 billion is fiduciary money and only about ½ a billion, primary money.

In the present chapter we shall exclude the consideration of bank deposits or check circulation and confine our attention to the circulation of money, primary and fiduciary. In the United States, the only primary money is gold coin. The fiduciary money includes (1) token coins, viz. silver dollars, fractional silver, and minor coins ("nickels" and cents); (2) paper money, viz. (a) certificates for gold and silver, and (b) promissory notes, whether of the United States government ("greenbacks"), or of the National banks.

Checks aside, we may classify exchanges into three groups: the exchange of goods against goods, or barter; the exchange of money against money, or changing money; and the exchange of money against goods, or purchase and sale. Only the last-named species of exchange makes up what we call the "circulation" of money. The circulation of money signifies, therefore, the aggregate amount of its transfers against goods. All money held for circulation, i.e. all money, except what is in the banks and United States government's vaults, is called "money in circulation."

The chief object of this book is to explain the causes determining the purchasing power of money. The purchasing power of money is indicated by the quantities of other goods which a given quantity of money will buy. The lower we find the prices of goods, the larger the quantities that can be bought by a given amount of money, and therefore the higher the purchasing power of money. The higher we find the prices of goods, the smaller the quantities that can be bought by a given amount of money, and therefore the lower the purchasing power of money. In short, the purchasing power of money is the reciprocal of the level of prices; so that the study of the purchasing power of money is identical with the study of price levels.

§ 2

Overlooking the influence of deposit currency, or checks, the price level may be said to depend on only three sets of causes: (1) the quantity of money in circulation; (2) its "efficiency" or velocity of circulation (or the average number of times a year money is exchanged for goods); and (3) the volume of trade (or amount of goods bought by money). The so-called "quantity theory,"*13 i.e. that prices vary proportionately to money, has often been incorrectly formulated, but (overlooking checks) the theory is correct in the sense that the level of prices varies directly with the quantity of money in circulation, provided the velocity of circulation of that money and the volume of trade which it is obliged to perform are not changed.

The quantity theory has been one of the most bitterly contested theories in economics, largely because the recognition of its truth or falsity affected powerful interests in commerce and politics. It has been maintained—and the assertion is scarcely an exaggeration—that the theorems of Euclid would be bitterly controverted if financial or political interests were involved.

The quantity theory has, unfortunately, been made the basis of arguments for unsound currency schemes. It has been invoked in behalf of irredeemable paper money and of national free coinage of silver at the ratio of 16 to 1. As a consequence, not a few "sound money men," believing that a theory used to support such vagaries must be wrong, and fearing the political effects of its propagation, have drifted into the position of opposing, not only the unsound propaganda, but also the sound principles by which its advocates sought to bolster it up.*14 These attacks upon the quantity theory have been rendered easy by the imperfect comprehension of it on the part of those who have thus invoked it in a bad cause.

Personally, I believe that few mental attitudes are more pernicious, and in the end more disastrous, than those which would uphold sound practice by denying sound principles because some thinkers make unsound application of those principles. At any rate, in scientific study there is no choice but to find and state the unvarnished truth.

The quantity theory will be made more clear by the equation of exchange, which is now to be explained.

The equation of exchange is a statement, in mathematical form, of the total transactions effected in a certain period in a given community. It is obtained simply by adding together the equations of exchange for all individual transactions. Suppose, for instance, that a person buys 10 pounds of sugar at 7 cents per pound. This is an exchange transaction, in which 10 pounds of sugar have been regarded as equal to 70 cents, and this fact may be expressed thus: 70 cents = 10 pounds of sugar multiplied by 7 cents a pound. Every other sale and purchase may be expressed similarly, and by adding them all together we get the equation of exchange for a certain period in a given community. During this same period, however, the same money may serve, and usually does serve, for several transactions. For that reason the money side of the equation is of course greater than the total amount of money in circulation.

The equation of exchange relates to all the purchases made by money in a certain community during a certain time. We shall continue to ignore checks or any circulating medium not money. We shall also ignore foreign trade and thus restrict ourselves to trade within a hypothetical community. Later we shall reinclude these factors, proceeding by a series of approximations through successive hypothetical conditions to the actual conditions which prevail to-day. We must, of course, not forget that the conclusions expressed in each successive approximation are true solely on the particular hypothesis assumed.

The equation of exchange is simply the sum of the equations involved in all individual exchanges in a year. In each sale and purchase, the money and goods exchanged are ipso facto equivalent; for instance, the money paid for sugar is equivalent to the sugar bought. And in the grand total of all exchanges for a year, the total money paid is equal in value to the total value of the goods bought. The equation thus has a money side and a goods side. The money side is the total money paid, and may be considered as the product of the quantity of money multiplied by its rapidity of circulation. The goods side is made up of the products of quantities of goods exchanged multiplied by their respective prices.

The important magnitude, called the velocity of circulation, or rapidity of turnover, is simply the quotient obtained by dividing the total money payments for goods in the course of a year by the average amount of money in circulation by which those payments are effected. This velocity of circulation for an entire community is a sort of average of the rates of turnover of money for different persons. Each person has his own rate of turnover which he can readily calculate by dividing the amount of money he expends per year by the average amount he carries.

Let us begin with the money side. If the number of dollars in a country is 5,000,000, and their velocity of circulation is twenty times per year, then the total amount of money changing hands (for goods) per year is 5,000,000 times twenty, or $100,000,000. This is the money side of the equation of exchange.

Since the money side of the equation is $100,000,000, the goods side must be the same. For if $100,000,000 has been spent for goods in the course of the year, then $100,000,000 worth of goods must have been sold in that year. In order to avoid the necessity of writing out the quantities and prices of the innumerable varieties of goods which are actually exchanged, let us assume for the present that there are only three kinds of goods,—bread, coal, and cloth; and that the sales are:—

200,000,000 loaves of bread at	$ .10 a loaf,
10,000,000 tons of coal at	5.00 a ton, and
30,000,000 yards of cloth at	1.00 a yard.

The value of these transactions is evidently $100,000,000, i.e. $20,000,000 worth of bread plus $50,000,000 worth of coal plus $30,000,000 worth of cloth. The equation of exchange therefore (remember that the money side consisted of $5,000,000 exchanged 20 times) is as follows:—

$5,000,000 × 20 times a year
	= 200,000,000 loaves × $ .10 a loaf
	+   10,000,000 tons    ×  5.00 a ton
	+   30,000,000 yards  ×  1.00 a yard.

This equation contains on the money side two magnitudes, viz. (1) the quantity of money and (2) its velocity of circulation; and on the goods side two groups of magnitudes in two columns, viz. (1) the quantities of goods exchanged (loaves, tons, yards), and (2) the prices of these goods. The equation shows that these four sets of magnitudes are mutually related. Because this equation must be fulfilled, the prices must bear a relation to the three other sets of magnitudes,—quantity of money, rapidity of circulation, and quantities of goods exchanged. Consequently, these prices must, as a whole, vary proportionally with the quantity of money and with its velocity of circulation, and inversely with the quantities of goods exchanged.

Suppose, for instance, that the quantity of money were doubled, while its velocity of circulation and the quantities of goods exchanged remained the same. Then it would be quite impossible for prices to remain unchanged. The money side would now be $10,000,000 × 20 times a year or $200,000,000; whereas, if prices should not change, the goods would remain $100,000,000, and the equation would be violated. Since exchanges, individually and collectively, always involve an equivalent quid pro quo, the two sides must be equal. Not only must purchases and sales be equal in amount—since every article bought by one person is necessarily sold by another—but the total value of goods sold must equal the total amount of money exchanged. Therefore, under the given conditions, prices must change in such a way as to raise the goods side from $100,000,000 to $200,000,000. This doubling may be accomplished by an even or uneven rise in prices, but some sort of a rise of prices there must be. If the prices rise evenly, they will evidently all be exactly doubled, so that the equation will read:—

$10,000,000 × 20 times a year
	= 200,000,000 loaves × $    .20 per loaf
	+   10,000,000 tons    ×   10.00 per ton
	+   30,000,000 yards  ×     2.00 per yard.

If the prices rise unevenly, the doubling must evidently be brought about by compensation; if some prices rise by less than double, others must rise by enough more than double to exactly compensate.

But whether all prices increase uniformly, each being exactly doubled, or some prices increase more and some less (so as still to double the total money value of the goods purchased), the prices are doubled on the average.*15 This proposition is usually expressed by saying that the "general level of prices" is raised twofold. From the mere fact, therefore, that the money spent for goods must equal the quantities of those goods multiplied by their prices, it follows that the level of prices must rise or fall according to changes in the quantity of money, unless there are changes in its velocity of circulation or in the quantities of goods exchanged.

If changes in the quantity of money affect prices, so will changes in the other factors—quantities of goods and velocity of circulation—affect prices, and in a very similar manner. Thus a doubling in the velocity of circulation of money will double the level of prices, provided the quantity of money in circulation and the quantities of goods exchanged for money remain as before. The equation will become:—

$5,000,000 × 40 times a year
	= 200,000,000 loaves × $    .20 a loaf
	+   10,000,000 tons    ×   10.00 a ton
	+   30,000,000 yards  ×     2.00 a yard,

or else the equation will assume a form in which some of the prices will more than double, and others less than double by enough to preserve the same total value of the sales.

Again, a doubling in the quantities of goods exchanged will not double, but halve, the height of the price level, provided the quantity of money and its velocity of circulation remain the same. Under these circumstances the equation will become:—

$5,000,000 × 20 times a year
	= 400,000,000 loaves × $  .05 a loaf
	+   20,000,000 tons    ×   2.50 a ton
	+   60,000,000 yards  ×     .50 a yard,

or else it will assume a form in which some of the prices are more than halved, and others less than halved, so as to preserve the equation.

Finally, if there is a simultaneous change in two or all of the three influences, i.e. quantity of money, velocity of circulation, and quantities of goods exchanged, the price level will be a compound or resultant of these various influences. If, for example, the quantity of money is doubled, and its velocity of circulation is halved, while the quantity of goods exchanged remains constant, the price level will be undisturbed. Likewise, it will be undisturbed if the quantity of money is doubled and the quantity of goods is doubled, while the velocity of circulation remains the same. To double the quantity of money, therefore, is not always to double prices. We must distinctly recognize that the quantity of money is only one of three factors, all equally important in determining the price level.

§ 3

The equation of exchange has now been expressed by an arithmetical illustration. It may be also represented visually, by a mechanical illustration. Such a representation is embodied in Figure 2. This represents a mechanical balance in equilibrium, the two sides of which symbolize respectively the money side and the goods side of the equation of exchange. The weight at the left, symbolized by a purse, represents the money in circulation; the "arm" or distance from the fulcrum at which this weight (purse) is hung represents the efficiency of this money, or its velocity of circulation. On the right side are three weights,—bread, coal, and cloth, symbolized respectively by a loaf, a coal scuttle, and a roll of cloth. The arm, or distance of each from the fulcrum, represents its price. In order that the lever arms at the right may not be inordinately long, we have found it convenient to reduce the unit of measure of coal from tons to hundredweights, and that of cloth from yards to feet, and consequently to enlarge correspondingly the numbers of units (the measure of coal changing from 10,000,000 tons to 200,000,000 hundred-weights, and that of the cloth from 30,000,000 yards to 90,000,000 feet). The price of coal in the new unit per hundredweight becomes 25 cents per hundredweight, and that of cloth in feet becomes 33 1/3 cents per foot.

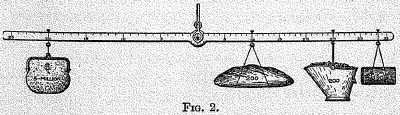
We all know that, when a balance is in equilibrium, the tendency to turn in one direction equals the tendency to turn in the other. Each weight produces on its side a tendency to turn, measured by the product of the weight by its arm. The weight on the left produces, on that side, a tendency measured by 5,000,000 × 20; while the weights on the right make a combined opposite tendency measured by 200,000,000×.10+200,000,000×.25+90,000,000×.33 1/3. The equality of these opposite tendencies represents the equation of exchange.

An increase in the weights or arms on one side requires, in order to preserve equilibrium, a proportional increase in the weights or arms on the other side. This simple and familiar principle, applied to the symbolism here adopted, means that if, for instance, the velocity of circulation (left arm) remains the same, and if the trade (weights at the right) remains the same, then any increase of the purse at the left will require a lengthening of one or more of the arms at the right, representing prices. If these prices increase uniformly, they will increase in the same ratio as the increase in money; if they do not increase uniformly, some will increase more and some less than this ratio, maintaining an average.

Likewise it is evident that if the arm at the left lengthens, and if the purse and the various weights on the right remain the same, there must be an increase in the arms at the right.

Again, if there is an increase in weights at the right, and if the left arm and the purse remain the same, there must be a shortening of right arms.

In general, a change in one of the four sets of magnitudes must be accompanied by such a change or change in one or more of the other three as shall maintain equilibrium.

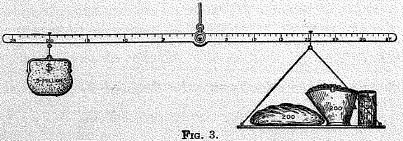
As we are interested in the average change in prices rather than in the prices individually, we may simplify this mechanical representation by hanging all the right-hand weights at one average point, so that the arm shall represent the average prices. This arm is a "weighted average" of the three original arms, the weights being literally the weights hanging at the right.

This averaging of prices is represented in Figure 3, which visualizes the fact that the average price of goods (right arm) varies directly with the quantity of money (left weight), and directly with its velocity of circulation (left arm), and inversely with the volume of trade (right weight).

§ 4

We now come to the strict algebraic statement of the equation of exchange. An algebraic statement is usually a good safeguard against loose reasoning; and loose reasoning is chiefly responsible for the suspicion under which economic theories have frequently fallen. If it is worth while in geometry to demonstrate carefully, at the start, propositions which are almost self-evident, it is a hundredfold more worth while to demonstrate with care the propositions relating to price levels, which are less self-evident; which, indeed, while confidently assumed by many, are contemptuously rejected by others.

Let us denote the total circulation of money, i.e. the amount of money expended for goods in a given community during a given year, by E (expenditure); and the average amount of money in circulation in the community during the year by M (money). M will be the simple arithmetical average of the amounts of money existing at successive instants separated from each other by equal intervals of time indefinitely small. If we divide the year's expenditures, E, by the average amount of money, M, we shall obtain what is called the average rate of turnover of money in its exchange for goods, E/M, that is, the velocity of circulation of money.*16 This velocity may be denoted by V, so that E/M = V; then E may be expressed as MV. In words: the total circulation of money in the sense of money expended is equal to the total money in circulation multiplied by its velocity of circulation or turnover. E or MV, therefore, expresses the money side of the equation of exchange. Turning to the goods side of the equation, we have to deal with the prices of goods exchanged and quantities of goods exchanged. The average*17 price of sale of any particular good, such as bread, purchased in the given community during the given year, may be represented by p (price); and the total quantity of it purchased, by Q (quantity); likewise the average price of another good (say coal) may be represented by p' and the total quantity of it exchanged, by Q'; the average price and the total quantity of a third good (say cloth) may be represented by p'' and Q'' respectively; and so on, for all other goods exchanged, however numerous. The equation of exchange may evidently be expressed as follows: *18—

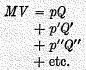
The right-hand side of this equation is the sum of terms of the form pQ—a price multiplied by a quantity bought. It is customary in mathematics to abbreviate such a sum of terms (all of which are of the same form) by using "S" as a symbol of summation. This symbol does not signify a magnitude as do the symbols M, V, p, Q, etc. It signifies merely the operation of addition and should be read "the sum of terms of the following type." The equation of exchange may therefore be written:—

That is, the magnitudes E, M, V, the p's and the Q's relate to the entire community and an entire year; but they are based on and related to corresponding magnitudes for the individual persons of which the community is composed and for the individual moments of time of which the year is composed.*19

The algebraic derivation of this equation is, of course, essentially the same as the arithmetical derivation previously given. It consists simply in adding together the equations for all individual purchases within the community during the year.*20

By means of this equation, MV = SpQ, the three theorems set forth earlier in this chapter may be now expressed as follows:—

(1) If V and the Q's remain invariable while M varies in any ratio, the money side of the equation will vary in the same ratio and therefore its equal, the goods side, must vary in that same ratio also; consequently, either the p's will all vary in that ratio or else some p's will vary more than in that ratio and others enough less to compensate and maintain the same average.*21

(2) If M and the Q's remain invariable while V varies in any ratio, the money side of the equation will vary in the same ratio, and therefore its equal, the goods side, must vary in that ratio also; consequently, the p's will all vary in the same ratio or else some will vary more and others enough less to compensate.

(3) If M and V remain invariable, the money side and the goods side will remain invariable; consequently, if the Q's all vary in a given ratio, either the p's must all vary in the inverse ratio or else some of them will vary more and others enough less to compensate.

We may, if we wish, further simplify the right side by writing it in the form PT where P is a weighted average of all the p's, and T is the sum of all the Q's. P then represents in one magnitude the level of prices, and T represents in one magnitude the volume of trade. This simplification is the algebraic interpretation of the mechanical illustration given in Figure 3, where all the goods, instead of being hung separately, as in Figure 2, were combined and hung at an average point representing their average price.

We have derived the equation of exchange, MV = SpQ, by adding together, for the right side, the sums expended by different persons. But the same reasoning would have derived an equation of exchange by taking the sums received by different persons. The results of the two methods will harmonize if the community has no foreign trade; for, apart from foreign trade, what is expended by one person in the community is necessarily received by some other person in that community.

If we wish to extend the reasoning so as to apply to foreign trade, we shall have two equations of exchange, one based on money expended and the other on money received or accepted by members of the community. These will always be approximately equal and may or may not be exactly equal within a country according to the "balance of trade" between that country and others. The right side of the equation based on expenditures will include, in addition to the domestic quantities already represented there, the quantities of goods imported and their prices, but not those exported; while the reverse will be true of the equation based on receipts.

§ 5

This completes our statement of the equation of exchange, except for the element of check payments, which is reserved for the next chapter. We have seen that the equation of exchange has as its ultimate basis the elementary equations of exchange pertaining to given persons and given moments, in other words, the equations pertaining to individual transactions. Such elementary equations mean that the money paid in any transaction is the equivalent of the goods bought at the price of sale. From this secure and obvious premise is derived the equation of exchange MV = SpQ, each element in which is a sum or an average of the like elementary elements for different individuals and different moments, thus comprising all the purchases in the community during the year. Finally, from this equation we see that prices vary directly as M and V, and inversely as the Q's, provided in each case only one of these three sets of magnitudes varies, and the other two remain unchanged. Whether to change one of the three necessarily disturbs the others is a question reserved for a later chapter. Those who object to the equation of exchange as a mere truism are asked to defer judgment until they have read Chapter VIII.

To recapitulate, we find then that, under the conditions assumed, the price level varies (1) directly as the quantity of money in circulation (M), (2) directly as the velocity of its circulation (V), (3) inversely as the volume of trade done by it (T). The first of these three relations is worth emphasis. It constitutes the "quantity theory of money."

So important is this principle, and so bitterly contested has it been, that we shall illustrate it further. As already indicated, by "the quantity of money" is meant the number of dollars (or other given monetary units) in circulation. This number may be changed in several ways, of which the following three are most important. Their statement will serve to bring home to us the conclusions we have reached and to reveal the fundamental peculiarity of money on which they rest.

As a first illustration, let us suppose the government to double the denominations of all money; that is, let us suppose that what has been hitherto a half dollar is henceforth called a dollar, and that what has hitherto been a dollar is henceforth called two dollars. Evidently the number of "dollars" in circulation will then be doubled; and the price level, measured in terms of the new "dollars," will be double what it would otherwise be. Every one will pay out the same coins as though no such law were passed. But he will, in each case, be paying twice as many "dollars." For example, if $3 formerly had to be paid for a pair of shoes, the price of this same pair of shoes will now become $6. Thus we see how the nominal quantity of money affects price levels.

A second illustration is found in a debased currency. Suppose a government cuts each dollar in two, coining the halves into new "dollars"; and, recalling all paper notes, replaces them with double the original number—two new notes for each old one of the same denomination. In short, suppose money not only to be renamed, as in the first illustration, but also reissued; prices in the debased coinage will again be doubled just as in the first illustration. The subdivision and recoinage is an immaterial circumstance, unless it be carried so far as to make counting difficult and thus to interfere with the convenience of money. Wherever a dollar had been paid before debasement, two dollars—i.e. two of the old halves coined into two of the new dollars—will now be paid instead.

In the first illustration, the increase in quantity was simply nominal, being brought about by renaming coins. In the second illustration, besides renaming, the further fact of recoining is introduced. In the first case the number of actual pieces of money of each kind was unchanged, but their denominations were doubled. In the second case, the number of pieces is also doubled by splitting each coin and reminting it into two coins, each of the same nominal denomination as the original whole of which it is the half, and by similarly redoubling the paper money.

For a third illustration, suppose that, instead of doubling the number of dollars by splitting them in two and recoining the halves, the government duplicates each piece of money in existence and presents the duplicate to the possessor of the original.*22 (We must in this case suppose, further, that there is some effectual bar to prevent the melting or exporting of money. Otherwise the quantity of money in circulation will not be doubled: much of the increase will escape.) If the quantity of money is thus doubled, prices will also be doubled just as truly as in the second illustration, in which there were exactly the same denominations. The only difference between the second and the third illustrations will be in the size and weight of the coins. The weights of the individual coins, instead of being reduced, will remain unchanged; but their number will be doubled. This doubling of coins must have the same effect as the 50 per cent debasement, i.e. it must have the effect of doubling prices.

The force of the third illustration becomes even more evident if, in accordance with Ricardo's presentation,*23 we pass back by means of a seigniorage from the third illustration to the second. That is, after duplicating all money, let the government abstract half of each coin, thereby reducing the weight to that of the debased coinage in the second illustration, and removing the only point of distinction between the two. This "seigniorage" abstracted will not affect the value of the coins, so long as their number remains unchanged.

In short, the quantity theory asserts that (provided velocity of circulation and volume of trade are unchanged) if we increase the number of dollars, whether by renaming coins, or by debasing coins, or by increasing coinage, or by any other means, prices will be increased in the same proportion. It is the number, and not the weight, that is essential. This fact needs great emphasis. It is a fact which differentiates money from all other goods and explains the peculiar manner in which its purchasing power is related to other goods. Sugar, for instance, has a specific desirability dependent on its quantity in pounds. Money has no such quality. The value of sugar depends on its actual quantity. If the quantity of sugar is changed from 1,000,000 pounds to 1,000,000 hundredweight, it does not follow that a hundredweight will have the value previously possessed by a pound. But if money in circulation is changed from 1,000,000 units of one weight to 1,000,000 units of another weight, the value of each unit will remain unchanged.

The quantity theory of money thus rests, ultimately, upon the fundamental peculiarity which money alone of all goods possesses,—the fact that it has no power to satisfy human wants except a power to purchase things which do have such power.*24